[最も選択された] taylor series expansion of log(1 x) about 0 344563

Jan 28, 16 · How can you find the taylor expansion of #ln(1x)# about x=0?In the denominator for each term in the infinite sumPlease be sure to answer the questionProvide details and share your research!

What Is The Correct Radius Of Convergence For Ln 1 X Mathematics Stack Exchange

Taylor series expansion of log(1 x) about 0

Taylor series expansion of log(1 x) about 0-Sep 03, 19 · Taylor series of log(x) with a = 1 Learn more about taylor, function, log(x), homeworkWrite down the Taylor series expansion, of the function log x about x = 1 upto three nonzero terms for x > 0

Upon Deriving Maclaurin S Series Expansion Of Tan X The Coefficients Are 1 16 272 7 936 37 594 What S The Pattern Among These Numbers Quora

Apr 15, 18 · 1 Taylor Series By M Bourne Our aim is to find a polynomial that gives us a good approximation to some function (See why we want to do this in the Introduction) We find the desired polynomial approximation using the Taylor Series If we want a good approximation to the function in the region near `x = a`, we need to find the first, second, third (and so on) derivativesBut avoid Asking for help, clarification, or responding to other answersFree math lessons and math homework help from basic math to algebra, geometry and beyond Students, teachers, parents, and everyone can find solutions to their math problems instantly

What is the Lagrange remainder for a ln(1x) Taylor series?View FOBAM FIN 234 vzomivggmyflrbpdf from FIN 234 at Bowling Green State University Find Taylor series at x=0 for the functions in Exercises 57â€"64 Step1 series expansion of about x = 0Free Taylor/Maclaurin Series calculator Find the Taylor/Maclaurin series representation of functions stepbystep This website uses cookies to ensure you get the best experience By using this website, you agree to our Cookie Policy

Dec 21,  · Earlier this semester, we saw how to approximate a function \(f (x, y)\) by a linear function, that is, by its tangent plane The tangent plane equation just happens to be the \(1^{\text{st}}\)degree Taylor Polynomial of \(f\) at \((x, y)\), as the tangent line equation was the \(1^{\text{st}}\)degree Taylor Polynomial of a function \(f(x)\)Calculus Power Series Constructing a Taylor Series 1 AnswerExpansions Which Have LogarithmBased Equivalents Summantion Expansion Equivalent Value Comments x n

Taylor Expansion Of Log X Page 1 Line 17qq Com

Taylor Expansion Of Log X Page 1 Line 17qq Com

Example 7 In this example we'll evaluate the harder limit lim x→0 cosx− 1 1 2 xsinx ln(1 x)4 Using Examples 2 and 4, lim x→0 cosx− 1 1 2 xsinx ln(1x)4 = limCompute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, historyMath 142 Taylor/Maclaurin Polynomials and Series Prof Girardi Fix an interval I in the real line (eg, I might be ( 17;19)) and let x 0 be a point in I, ie, x 0 2I

Taylor Series Wikipedia

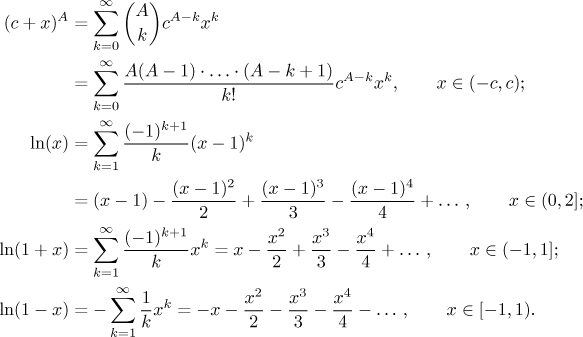
Math Tutor Series Methods Survey Function Series

> 1 Taylor polynomials > 11 The Taylor polynomial Example Find a quadratic polynomial p 2(x) to approximate f(x) near x= a Since p 2(x) = b 0 b 1xb 2x2 we impose three conditions on p 2(x) to determine the coefficientsTo better mimic f(x) at x= awe requireTaylor Series Expansion of cos x and sin x in C Programming without using mathh and only inside int main() 2 Verilog code to compute cosx using Taylor series approximation77 EXPANSION RELIEF VALVES Taylor 7700 Series, 1" Cl150 x 1" Cl 150 RF, Tee Body, Trim 174 H900 W/ Viton Seats, CS Body, Spring Range (Set point must be provided at time of order) $

What Is The General Term For E X 1 X Mathematics Stack Exchange

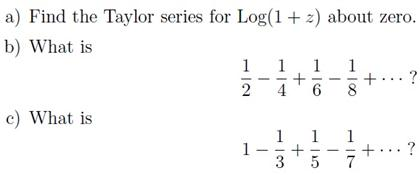
Solved Find The Taylor Series For Log 1 Z About Zero Chegg Com

Local polynomial approximation through Taylor series Value Vector of length n1 representing a polynomial of degree n Details Calculates the first four coefficients of the Taylor series through numerical differentiation and uses some polynomial `yoga'Find a Taylor series expansion for {eq}\ln(12x) {/eq} centered at {eq}c=0 {/eq} using the formula for a geometric sequence Finding Taylor Series for Logarithmic Function First, we are given aHe states the following we can expand $\ln\Omega(EE_v)$ in the Taylor series $$\ln\Omega(EE_v) = \ln\Omega(E) Stack Exchange Network Stack Exchange network consists of 176 Q&A communities including Stack Overflow , the largest, most trusted online community for developers to learn, share their knowledge, and build their careers

How To Expand Log 1 Log 1 X In Powers Of X As Far As X 4 Quora

Expand Log 1 Sinx Up To The Term Containing X 4 By Using Maclaurin S Theorem Sarthaks Econnect Largest Online Education Community

Jan 31, 18 · Let's say we wanted a Taylor series approximation for ln(1 x) about a = 2 Then, the series will converge for the values of x within the interval of convergence The lefthand point is 1X3 00 (12) ≈ 1x− 1 3 x3, (13) and this last line is indeed the first three terms of the Taylor series of cos(x)ex about x = 0, as you can verify tediously and directly via Eq (6) Note how there was a funny cancellation leading to no quadratic term x2 in the answer Eq (13) The key step for you to pay attention to is in line EqGet the free "Log(1x) Taylor Series" widget for your website, blog, Wordpress, Blogger, or iGoogle Find more Mathematics widgets in WolframAlpha

Taylor Series Wikipedia

Maclaurin Series For Ln 1 X Youtube

About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy & Safety How works Test new features Press Copyright Contact us CreatorsIn this blog, I want to review famous Taylor Series Expansion and its special case Maclaurin Series Expansion According to wikipedia, the aim of Taylor Series Expansion (TSE) is to represent a function as an infinite sum of terms that are derived from the values of that function's derivatives, which in turn are evaluated at some predefined single pointTaylor Series Calculator with Steps Taylor Series, Laurent Series, Maclaurin Series Enter a, the centre of the Series and f(x), the function See Examples

Maclaurin Series For Ln 1 X How To Steps Video Lesson Transcript Study Com

Upon Deriving Maclaurin S Series Expansion Of Tan X The Coefficients Are 1 16 272 7 936 37 594 What S The Pattern Among These Numbers Quora

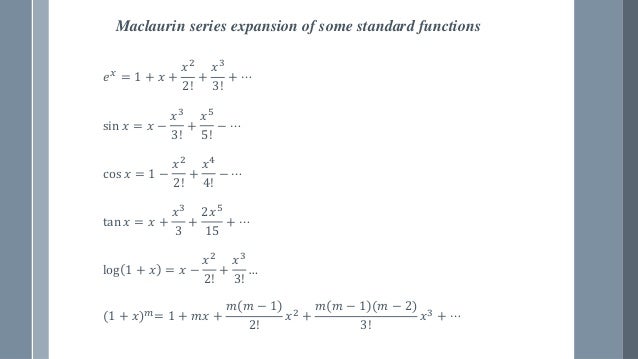
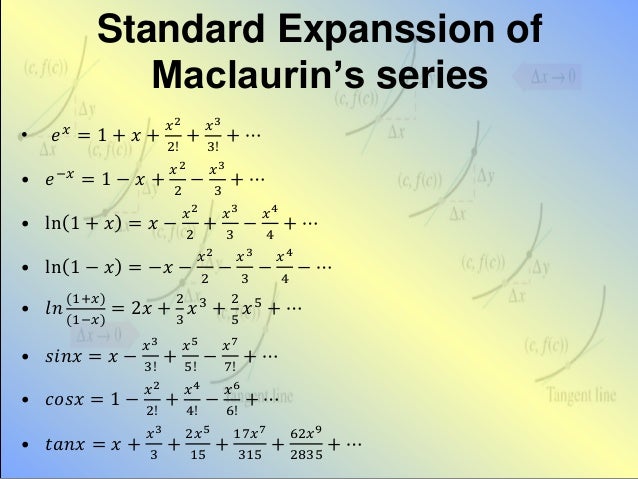
May 26,  · In this section we will discuss how to find the Taylor/Maclaurin Series for a function This will work for a much wider variety of function than the method discussed in the previous section at the expense of some often unpleasant work We also derive some well known formulas for Taylor series of e^x , cos(x) and sin(x) around x=0Thanks for contributing an answer to Mathematics Stack Exchange!A Taylor Series is an expansion of some function into an infinite sum of terms, where each term has a larger exponent like x, x 2, x 3, etc Example The Taylor Series for e x e x = 1 x x 2 2!

Write The Maclaurin Series Expansion Of The Following Functions I E X Ii Sin X Iii Cos X Iv Log 1 X 1 X 1 Sarthaks Econnect Largest Online Education Community

Series Expansion Of Exponential And Logarithmic Functions

Using 0th order Taylor series ex ˇ1 does not give a good fit Using 1st order Taylor series ex ˇ1 x gives a better fit Using 2nd order Taylor series ex ˇ1 x x2=2 gives a a really good fit 1 importnumpy as np 2 x = 3 pn = 00 4 forkinrange(15) 5 pn = (x**k) / mathfactorial(k) 6 err = npexp() pn 6The power series expansion for f( x) can be differentiated term by term, and the resulting series is a valid representation of f′( x) in the same interval Differentiating again gives and so on Substituting and in general, substituting x = 0 in the power series expansion for the nth derivative of f yields These are called the Taylor coefficients of f, and the resulting power seriesTopic Finding the Taylor Series of the Log(1x) or Log(1x) using the Geometric Series What you should know?

Solved We Can Approximate The Natural Logarithm Using A T Chegg Com

Power Series Taylor S And Maclaurin S Series

A Taylor series approximation uses a Taylor series to represent a number as a polynomial that has a very similar value to the number in a neighborhood around a specifiedWith PythonNumpy, it's probably optimized here and there, and so it's impossible to really benchmark log(1x) vs x 05 * x^2 So I moved to C Result Time per operation with log 1957 ns Time per operation with order2 Taylor expansion of log 373 ns So roughly a x5 factor !Jan 02, 17 · Taking the derivative of the MacLaurin series gives you $1 x x^2 x^3 x^4 \ldots$ Since this is a geometric series with ratio $x$, it equals $\frac{1}{1 x}$ when x is in $(1, 1)$ This shows the expression $\ln(1x)$ and its MacLaurin expansion to have the same derivative over $(1, 1)$, which means they are equal within a constant

Maclaurin Series Of Eˣ Video Khan Academy

The Natural Logarithm And Its Series Expansion 2 Ways Ln X 1 At 0 Youtube

The calculator will find the Taylor (or power) series expansion of the given function around the given point, with steps shown You can specify the order of the Taylor polynomial If you want the Maclaurin polynomial, just set the point to `0`T = taylor(f,var) approximates f with the Taylor series expansion of f up to the fifth order at the point var = 0If you do not specify var, then taylor uses the default variable determined by symvar(f,1)Taylor series expansions of logarithmic functions and the combinations of logarithmic functions and trigonometric, inverse trigonometric, hyperbolic, and inverse hyperbolic functions

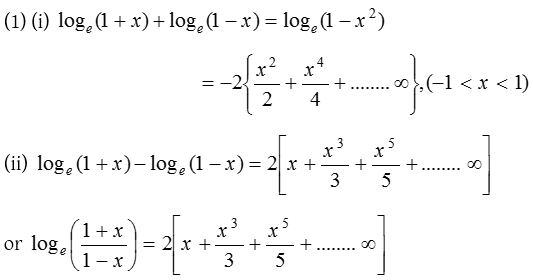
What Is Logarithmic Series Expansion A Plus Topper

Taylor Series Wikipedia

Taylor series of a function is the sum of infinite series or infinite terms Taylor series is polynomial of sum of infinite degree It is used in various fields such as calculus Maclaurin Series ExpansionPhysics 116A Winter 11 Taylor Series Expansions In this short note, a list of wellknown Taylor series expansions is provided We focus on Taylor series about the point x = 0, the socalled Maclaurin seriesIf you know Lagrange's form of the remainder you should not need to ask Also you haven't said what point you are expanding the function about (although it must be greater than )

Taylor Series Wikipedia

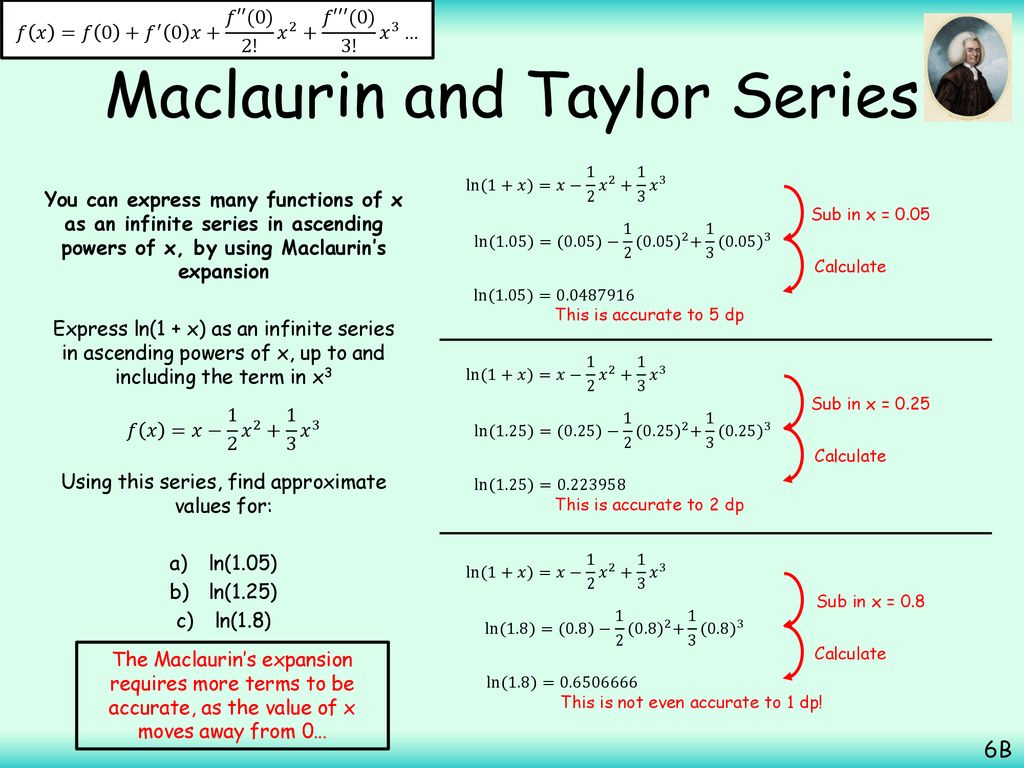
Maclaurin And Taylor Series Ppt Download

The result 70 is the same as the result we calculated when we wrote out each term of the Taylor Series individually An advantage of using a for loop is that we can easily increase the number of terms If we increase the number of times the for loop runs, we increase the number of terms in the Taylor Series expansion Let's try 10 terms Note how the line for i in range(10) now includes 10Geometric Series Integration of power functDec 29,  · Since the Taylor series for \(\sin x\) has an infinite radius of convergence, so does the Taylor series for \(\sin(x^2)\) The Taylor expansion for \(\ln x\) given in Key Idea 32 is centered at \(x=1\),so we will center the series for \(\ln (\sqrt{x})\) at \(x=1\) as well With

How To Expand Log 1 Log 1 X In Powers Of X As Far As X 4 Quora

Logarithms Logs Log Ln Lg

Jan 14, 15 · The case of particular interest is the expansion of function in the vicinity of x=0 Such series is called MacLaurin expansion, and that's what we're going to consider for the cases of some common functions Of course, this only refers to the vicinity of the point x_0=0 Often in Taylor series related homework tasks you'll faceAnd more generally, the corresponding Taylor series for log(x) at some is The Taylor series for the exponential function ex at a = 0 is The above expansion holds because the derivative of e xwith respect to x is also e and e0 equals 1 This leaves the terms (x − 0)n in the numerator and n!Summary The taylor series calculator allows to calculate the Taylor expansion of a function taylor_series_expansion online Description The online taylor series calculator helps determine the Taylor expansion of a function at a point The Taylor expansion of a function at a point is a polynomial approximation of the function near that point

Logarithms Logs Log Ln Lg

What Is The Correct Radius Of Convergence For Ln 1 X Mathematics Stack Exchange

Dec 29, 14 · Logarithms of Taylor Expansions I've already described how Herbie computes series expansions to produce polynomial approximations to floatingpoint programs There, I described how expansions of exponentials and the trigonometric functions worked, but I didn't have a way to expand logarithms and thus powersThe Taylor series of a function is extremely useful in all sorts of applications and, at the same time, it is fundamental in pure mathematics, specifically in (complex) function theory Recall that, if f (x) f(x) f (x) is infinitely differentiable at x = a x=a x = a, the Taylor series of f (x) f(x) f (x) at x = a x=a x = a is by definitionF0(z) = X1 n=0 na n(z z 0)n 1 The series for f0also has radius of convergence R 4If is a bounded curve inside the disk of convergence then the integral is given by termbyterm integration Z f(z)dz= X1 n=0 Z a n(z z 0)n Notes The theorem doesn't say what happens when jz z 0j= R If R= 1the function f(z) is entire

Madhav University Maclaurin S Theorem

Expansion Of Logd Page 1 Line 17qq Com

Series Expansion For E Sin X And Coefficient Comparison Mathematics Stack Exchange

Natural Logarithm Wikipedia

Answered X X3 Log 1 X X 2 3 4 For 1 Bartleby

Expand Log 1 Sinx Up To The Term Containing X 4 By Using Maclaurin S Theorem Sarthaks Econnect Largest Online Education Community

Write The Maclaurin Series Expansion Of The Following Functions I E X Ii Sin X Iii Cos X Iv Log 1 X 1 X 1 Sarthaks Econnect Largest Online Education Community

Maclaurin And Taylor Series Power Series Expansion Of Logarithmic Function

Taylor Expansion Of Log X Page 1 Line 17qq Com

Taylor Series Wikipedia

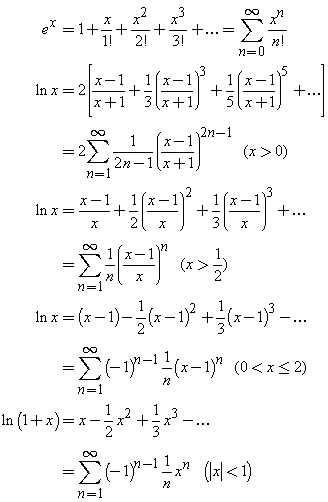
Series Expansions Of Exponential And Logarithmic Functions

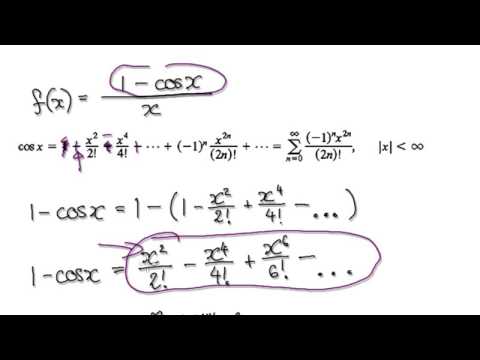
Video 2776 Maclaurin Series 1 Cosx X Practice Youtube

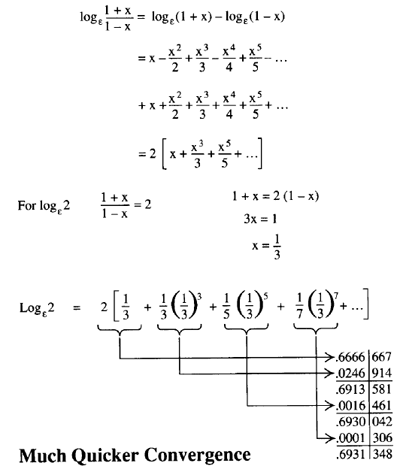
Logarithms Logs Log Ln Lg

Taylor Series Wikipedia

Taylor Series Wikipedia

F A X 4 Expand The Following Functions In A Maclaurin Series 2 1 X B 1 Homeworklib

Maclaurin Series Of Eˣ Video Khan Academy

Expand Log X In Powers Of X 1 By Taylor S Series Sarthaks Econnect Largest Online Education Community

Expand 1 Dim Vector By Using Taylor Series Of Log 1 E X In Python Stack Overflow

Series Expansion Of Exponential And Logarithmic Functions

Finding A Maclaurin Series Expansion Another Example 1 Youtube

Expand Log 1 E X In Ascending Powers Of X Up To The Term Containing X 4 Sarthaks Econnect Largest Online Education Community

Maclaurin Series For Ln 1 X How To Steps Video Lesson Transcript Study Com

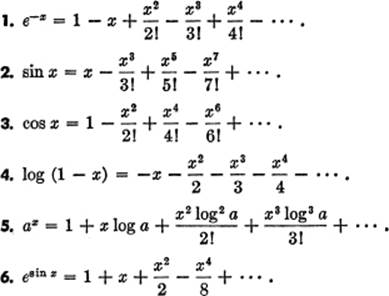
Expansion Of Functions Expansion Of Functions The Calculus Primer

Expand Log Sinx In Powers Of X 2 By Taylor S Theorem Youtube

Taylor Series Wikipedia

Expansion Of Log 1 Sinx Youtube

Write The Maclaurin Series Expansion Of The Following Functions I E X Ii Sin X Iii Cos X Iv Log 1 X 1 X 1 Sarthaks Econnect Largest Online Education Community

By Using Maclaurin S Theorem Expand Log Sec X Up To The Term Containing X 6 Sarthaks Econnect Largest Online Education Community

Expand 1 Dim Vector By Using Taylor Series Of Log 1 E X In Python Stack Overflow

Help With Arithmetic On Basic Taylor Series Expansion Mathematics Stack Exchange

Expansion Of Functions Expansion Of Functions The Calculus Primer

Expand Log Sinx In Powers Of X 2 By Taylor S Theorem Maths Differential Equations Meritnation Com

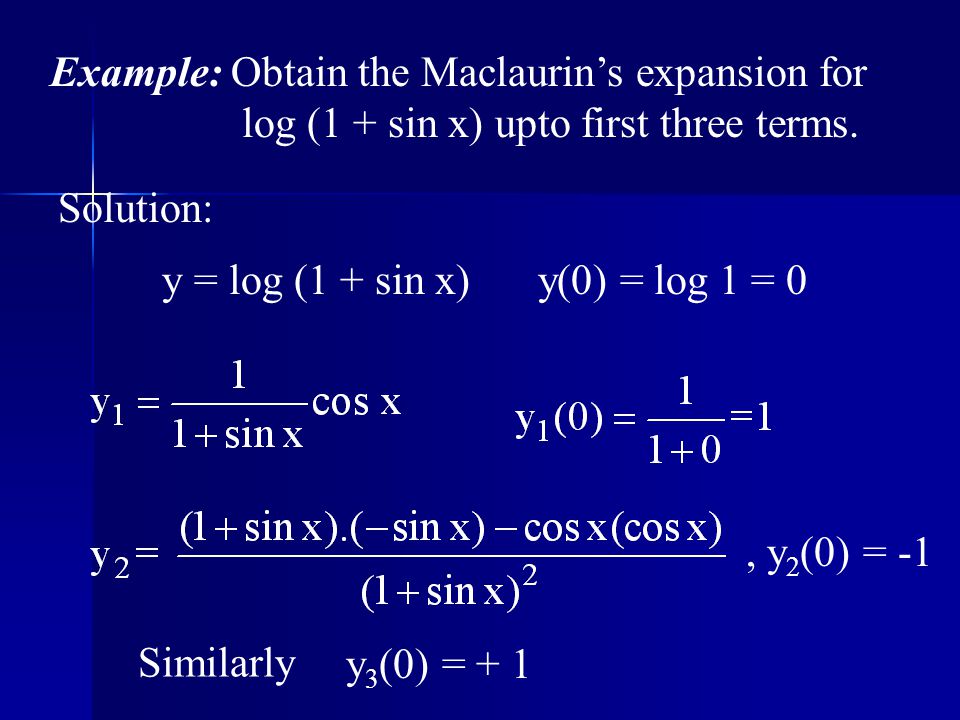
Example Obtain The Maclaurin S Expansion For Ppt Video Online Download

What Is The Taylor Series Expansion Of Math E X Math About Zero Quora

Expand Log 1 X Y Up To Third Degree Terms About The Origin Sarthaks Econnect Largest Online Education Community

Write Down The Taylor Series Expansion Of The Function Log X About X 1 Upto Three Non Zero Terms For X 0 Sarthaks Econnect Largest Online Education Community

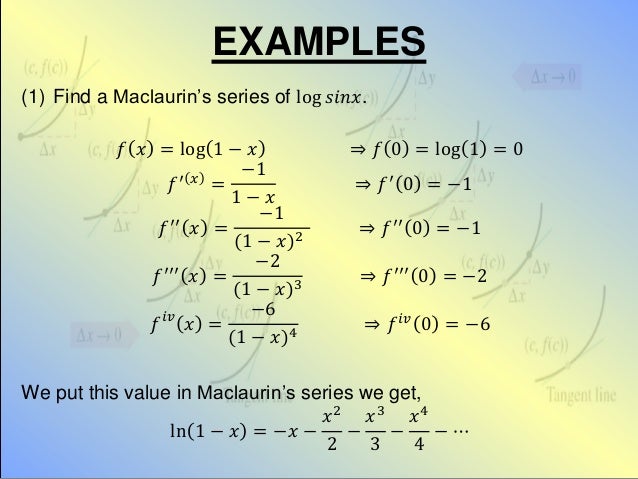
Taylor S Maclaurin S Series Simple

What Is The Taylor Series Expansion Of Sin X Quora

Taylor Series Expansions Of Logarimathic Functions

Log X Maclaurin Series Page 1 Line 17qq Com

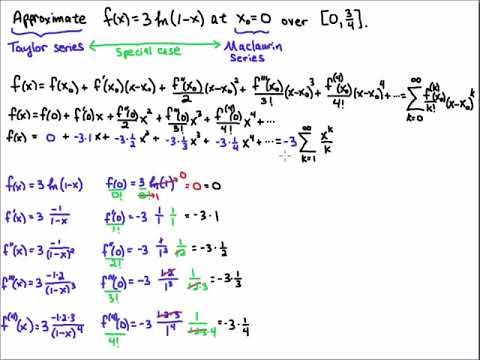
Taylor Series Expansion Of Natural Log Function Youtube

Taylors And Maclaurins Series Mathematical Relations Mathematical Concepts

Expansion Of Log 1 X Maclaurin Series Youtube

Taylor Series Wikipedia

Natural Logarithm Series

Taylor Series For Log X Physics Forums

How Do You Do The Taylor Expansion For F X Log X 1 At X 0 Socratic

Expand E X Log 1 Y By Maclaurin S Theorem Up To The Third Degree Term Sarthaks Econnect Largest Online Education Community

Taylor Series Wikipedia

Expand Logex In Power Of X And Hence Evaluate Loge 1 1 Correct To Four Decimal Places Answer Mathematics 1 Question Answer Collection

Expand Log 1 E X In Ascending Powers Of X Up To The Term Containing X 4 Sarthaks Econnect Largest Online Education Community

Log 1 E X Maclaurin Series

Solved It Is A Fact That The Taylor Series Expansion Aro Chegg Com

Cochranmath Taylor Series Integration And Differentiation

Maclaurin And Taylor Series Power Series Expansion Of Logarithmic Function

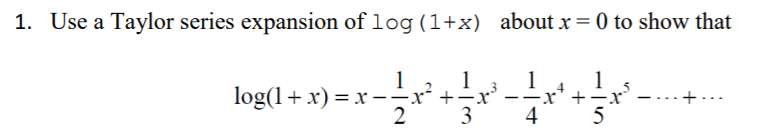
Solved 1 Use Taylor Series Expansion Log 1 X Aboutx 0 Show Q

Example Obtain The Maclaurin S Expansion For Ppt Video Online Download

Solved In 1 X Can Be Approximated Using The Following Chegg Com

Example Obtain The Maclaurin S Expansion For Ppt Video Online Download

Expansion Of Sinhx Using Maclaurin S Series Expansions Of Functions Engineering Mathematics 1 Youtube

Taylor Series Wikipedia

Graphs Of Taylor Polynomials Wolfram Demonstrations Project

Maclaurin Series For Ln 1 X How To Steps Video Lesson Transcript Study Com

What Is The Expansion Of Log 1 X Quora

9 Maclaurin S Theorem Problem 1 Differential Calculus Youtube

Power Series Taylor S And Maclaurin S Series

Natural Logarithm Wikipedia

Taylor Series For Log X Physics Forums

I Am Taking A Taylor Series Expansion Of A Function F X I Wish To Expand The Function Around The Value G X Is This Allowable

Taylor Series For Ln 1 X How To Steps Math Class 21 Video Study Com